top of page


A t-test is an inferential test used to compare the means of two populations. There are several different kinds of t-tests. This page will discuss the independent samples t-test. Other types of t-tests are: one sample and paired samples.

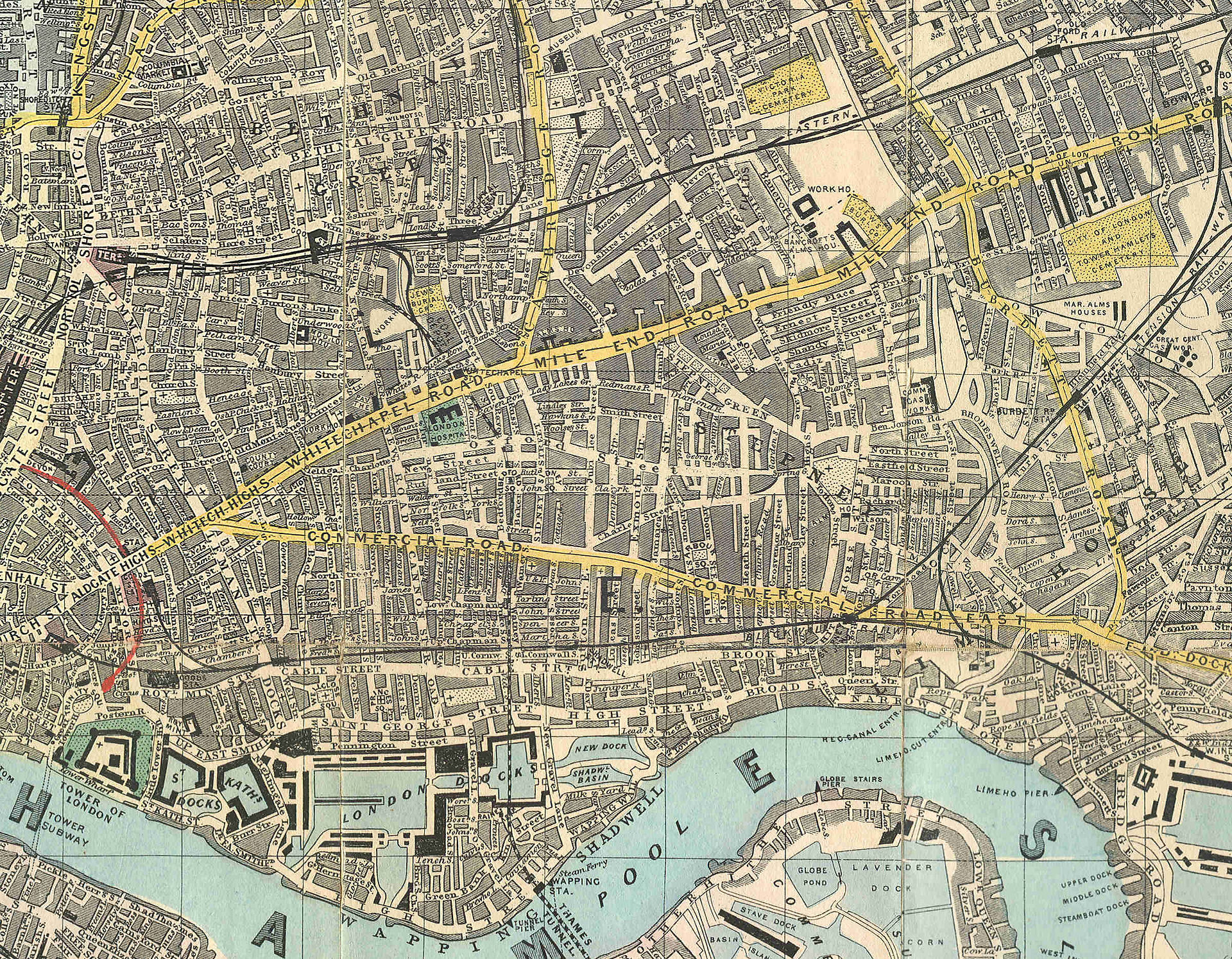
The data for this example is anonymized data from the most recent benchmark exam taken by approximately 200 students at my school. For the purposes of this illustration, I selected one class period from my students and one class period from my colleague to compare the sample means of the two groups. I was interested to see if the sample mean of the percentage of correct answers on the benchmark had a statistically significant difference.



An independent samples t-test was conducted to compare the percentage of correct answers on the March Benchmark Exam in Shull’s class and Harwell’s class conditions. There was no significant difference in the scores for Shull’s class (M=51.67, SD=16.17) and for Harwell’s class (M=49.02, SD=18.38) conditions; t(50)=0.554, p=0.582. There is not enough evidence to reject the null hypothesis that there is no significant difference between Shull’s class and Harwell’s class.
Salkind, N. J. (2017) Statistics for people who (think they) hate statistics. Thousand Oaks, CA: SAGE Publications
bottom of page